Explanation of a Ball Lenses | Glass Ball Lenses | Sapphire Ruby Ball Lenses
Ball lenses are great optical components for improving signal coupling between fibers, emitters, and detectors. They are also used in endoscopy, bar code scanning, ball pre-forms for aspheric lenses, and sensor applications. Ball lenses are manufactured from a single substrate of glass and can focus or collimate light, depending upon the geometry of the input source. Half-ball lenses are also common and can be interchanged with full ball lenses if the physical constraints of an application require a more compact design.
VY Optics offers a variety of ball lenses and half ball lens in a range of substrates for performance in the ultraviolet to the NIR.
Material options include: quartz (260-2500nm), sapphire (0.17–5.5 μm), ruby (250 nm to NIR), BK7 (or equivalent) (250–2500 nm), UV-grade fused silica (170–2500 nm), as well as B270 (320–2600 nm) and borosilicate (or equivalent) (310–2700 nm) material options etc.
Essential Equations for Using Ball Lenses
There are five key parameters needed to understand and use ball lenses (Figure 1): Diameter of Input Source (d), Diameter of Ball Lens (D), Effective Focal Length of Ball Lens (EFL), Back Focal Length of Ball Lens (BFL) and Index of Refraction of Ball Lens (n).

EFL is very simple to calculate (Equation 1) since there are only two variables involved: Diameter of Ball Lens (D) and Index of Refraction (n). EFL is measured from the center of the ball lens, indicated by R in Figure 1. BFL (Equation 2) is easily calculated once EFL and D are known.
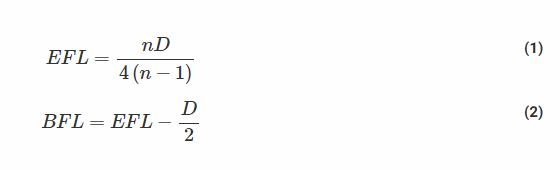
For collimated incident light, the numerical aperture (NA) of the ball lens is dependent on the size of the ball lens (D), its index of refraction (n), and the diameter of the input source (d). Using f-number = EFL/d, a relation between NA and d/D can be obtained (Equation 3), which is plotted in Figure 2.
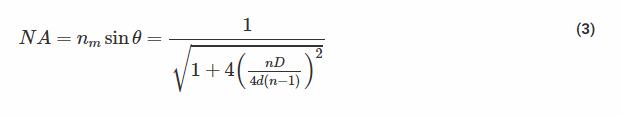
Equation 3 assumes that the refractive index outside of the ball lens (nm) equals 1. In the paraxial limit (i.e. d/D<<1), the numerical aperture can be estimated from the f-number as NA ≈ 1/(2 x f-number), which yields Equation 4.


As d/D increases, the focused spot size at the back focal length of the lens increases, due to increased spherical aberration.
Application Examples
Example 1: Laser to Fiber Coupling
When coupling light from a laser into a fiber optic, the choice of ball lens is dependent on the NA of the fiber and the diameter of the laser beam, or the input source. The diameter of the laser beam is used to determine the NA of the ball lens. The NA of the ball lens must be less than or equal to the NA of the fiber optic in order to couple all of the light. The ball lens is placed at its back focal length from the fiber as shown in Figure 3.
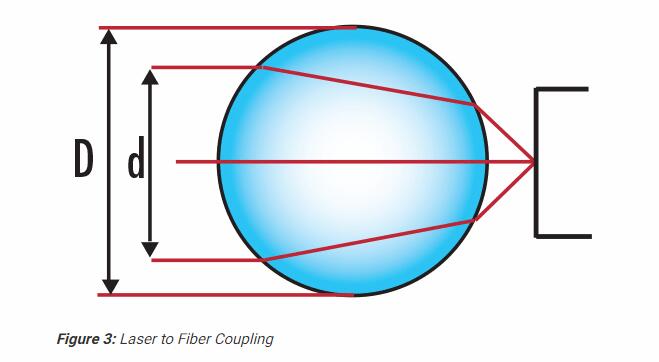
Initial Parameters
Diameter of Input Laser Beam = 2mm
Index of Refraction of Ball Lens = 1.5168
Numerical Aperture of Fiber Optic = 0.22
From Figure 2, the NA of an N-BK7 ball lens is about 0.22 for d/D ≈ 0.3 to 0.35. Equation 3 yields d/D ≈ 0.33 for NA = 0.22. You would need an N-BK7 ball lens with a diameter greater than 6mm (≈ 2mm/0.33) to couple a 2mm laser source into a 0.22 NA fiber optic. One can easily try different indices of refraction in order to find the best ball lens for a laser-to-fiber coupling application.
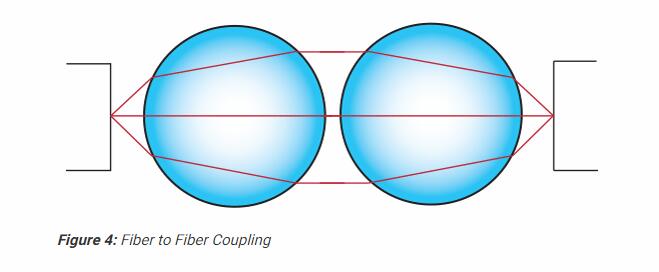
Example 2: Fiber to Fiber Coupling
To couple light from one fiber optic to another fiber optic of similar NA, two identical ball lenses can be used. Place the two ball lenses at the back focal length from the fibers as shown in Figure 4. If the optical fibers have the same NA, then the same logic as in Example 1 can be applied.